How To Prove Congruent Triangles Examples
AAS Postulate angle angle side Two equal angles and a side that does not lie between the two angles prove that a pair of triangles are congruent by the AAS Postulate Angle Angle Side. Sometimes when you are trying to decide if triangles are congruent you need to identify other sides or angles that are congruent.
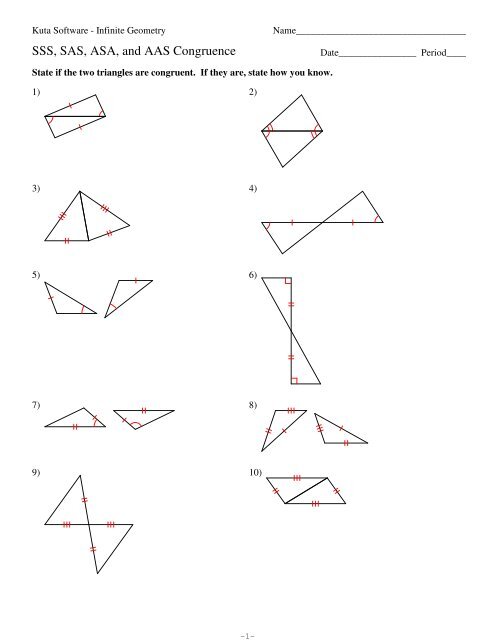
Geo Chapter 4 Lesson 2 Homework Congruent Triangle Theorems Geometry Worksheets Congruent Triangles Worksheet Math Geometry
Two triangles ABC and PQR are such that.

How to prove congruent triangles examples. Learn how to use the Triangle Proportionality Theorem to complete triangle proportions solve word problems and find the value of the missing sides of a triangle. Below is the proof that two triangles are congruent by Side Angle Side. Triangle Congruence Theorems file name.
AB 35 cm BC 71 cm AC 5 cm PQ 71 cm QR 5 cm and PR 35 cm. Complete videos list. When triangles are congruent all pairs of corresponding sides are congruent and all pairs of corresponding angles are congruent.
In Step 1 Sal stated that angles AEB and DEC are congruent because they are vertical angles. Check whether the triangles are congruent. Examples solutions videos and lessons to help High School students learn how to use congruence and similarity criteria for triangles to solve problems and to prove relationships in geometric figures.
SideSide-Side SSS If AB EF BC FG AC EG then ΔABC ΔEFG. Congruent by what we abreviate to be CPCTC which means Corresponding Parts of Congruent Triangles are Congruent. A Name four pairs of vertical angles.
Triangle ABC and PQR are congruent ABC PQR if length BAC PRQ ACB PQR. 8 7 6 5 4 3 2 1 Name. Proving that a point is the midpoint via triangle congruencyWatch the next lesson.
SideAngleSide SAS If AB EF BAC FEG AC EG then ΔABC ΔEFG. Fortunately it is not necessary to show all six of these facts to prove triangle congruence. If the hypotenuse and one leg of a right triangle are equal to the hypotenuse and one leg of another right triangle then the two right triangles are congruent.
In the right triangles ΔABC and ΔPQR if AB PR AC QR then ΔABC ΔRPQ. Vertical angles are angles that across from each other and made by two intersecting lines and they are ALWAYS congruent. HSG-SRTB5 CPCTC is an acronym for Corresponding Parts of Congruent Triangles are Congruent.
In the example of the frame of an umbrella at the right we can prove the two triangles congruent by SAS. Worked examples of triangle congruence. Can you imagine or draw on a piece of paper two triangles B C A X C Y whose diagram would be consistent with the Side Angle Side proof shown below.
The five ways of identifying congruent triangles are shown below. Figure 4 Heading. This article includes the Triangle Proportionality Theorem proof and examples that can help you fully gauge your understanding of it.
Again you have to prove the two triangle congruent before you can ever use CPCTC. AB PR 35 cm. B Name four pairs of corresponding angles.
The Hypotenuse-Leg HL Rule states that. _____ Unit 8 Day 3 - Proving Triangles Congruent Classwork 1. Proving Triangles Congruence Rules Theorems.
If two triangles have one angle equal and two sides on either side of the angle equal the triangles are congruent by SAS Postulate Side Angle Side. There are five ordered combinations of these six facts that can be used to prove triangles congruent.
Read more »