Property Of Equality In Mathematics
Subtraction property of equality. Anyway just defining equality to mean another word or phrase which is synonymous with equality isnt going to get us anywhere.
Properties Of Equality Png 648 380 Addition Words Sat Math Rational Numbers
That is we can interchange the sides of.

Property of equality in mathematics. The properties of equality are. Divide both sides by 4. Division Property Of Equality Formula.
It is denoted by the symbol which always goes between these two objects. Addition and subtraction or. The division property of equality states that when we divide both sides of an equation by the same non-zero number the two sides remain equal.
An inverse operation are two operations that undo each other eg. The symmetric property of equality is one of the basic properties of equality in mathematics. This property states that multiplying or dividing both sides of an equation by the same nonzero number produces an equivalent equationan equation that has the same solution.
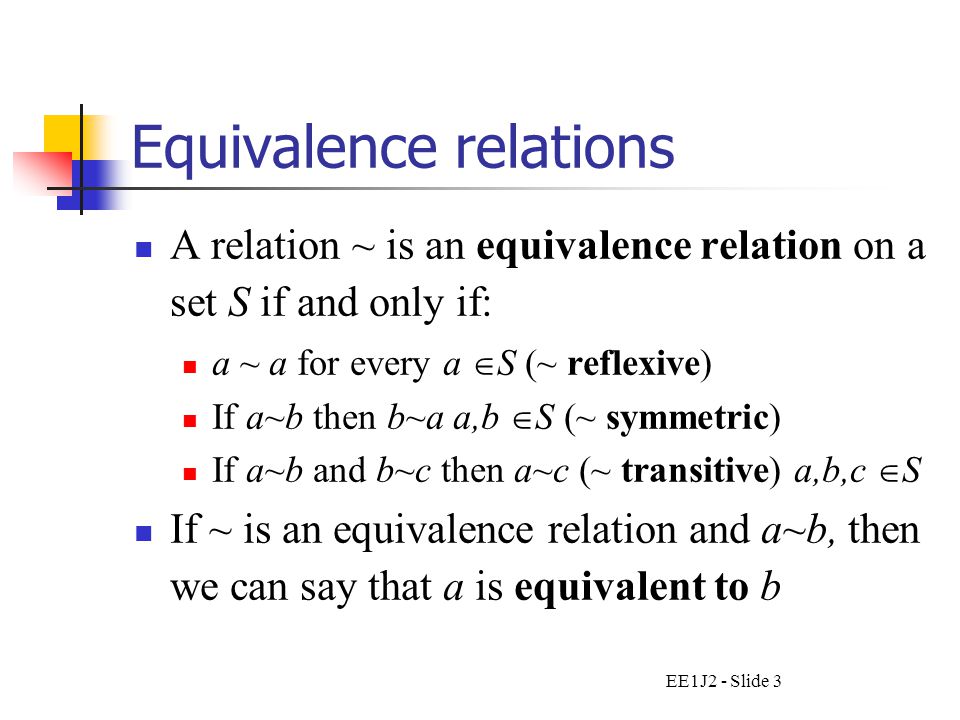
Ultimately my impression is that you might just assume that equality is an equivalence relation when setting up mathematics via first. Free Algebraic Properties Calculator - Simplify radicals exponents logarithms absolute values and complex numbers step-by-step. Others include the reflexive and transitive properties of equality.
The Division Property of Equality states. This is very similar to the multiplication property of equality in which we can multiply both sides of any equation without affecting the equation. The multiplication property of equality states that when we multiply both sides of an equation by the same number the two sides remain equal.
I f a b t h e n a c b c. A number equals. Property of equality along with other properties from algebra such as the distributive property a b c ab ac can be used to solve equations.
In another word that two objects are the same thing. For instance let us solve the equation given below. Multiplication property of equality.
Addition property of equality. Division property of equality. In mathematics the symmetric property of equality is really quite simple.
The symmetric property of equality states that for two variables a and b. For any real numbers a b and c where c does not equal 0 if a b then a c b c and c b c a. For all real numbers x x x.
Multiplication Property of Equality. That is if a b and c are real numbers such that a b and c 0 then a c a c. That is if a b and c are real numbers such that a b then a c b c Example 1.
Lisa and Linda have got the same amount of money. If a b then b a. Therefore multiplying or dividing the.
This expression is used to establish that two mathematical objects represent the same object. The transitive property of equality states that for any quantities a b and c if a is equal to b and b is equal to c then a must be equal to c. And this as we learned in a previous section is shown by the equality sign.
Properties of equalities Two equations that have the same solution are called equivalent equations eg. 9 rows PROPERTIES OF EQUALITY. 5 3 2 6.
This property states that if a b then b a. If a b and b c then a. We use this property to transform an equation into a simpler one.
Consider the equation 12 12. The properties of equality they refer to the relationship between two mathematical objects either numbers or variables.
Read more »Labels: mathematics, property