PRORFETY: What Does Transitive Property Mean In Geometry

Transitive Property of Inequalities. If a b a b and b c b c then a c a c.

Math Properties Transitive Property Of Inequality

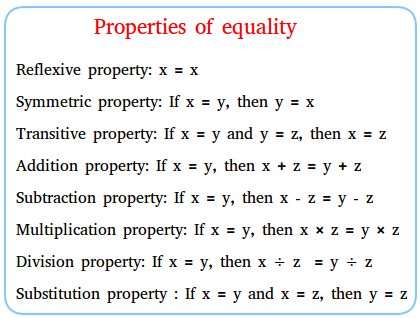
The Transitive Property states that for all real numbers x y and z if x y and y z then x z.

What does transitive property mean in geometry. Transitivity requires that if a b and b c are present in the relation then so is a c. Being a child is a transitive relation being a parent is not. If a b and b c then a c.

The transitive property of congruence states that two objects that are congruent to a third object are also congruent to each other. This can be tricky to explain so we will use examples. If aband bc then ac.

The transitive property of inequality also holds true for less than greater than or equal to and less than or equal to. Definition In this video lesson we talk about the transitive property of equality. Pictures and examples explaining the most frequently studied math properties including the associative distributive commutative and substitution property.

The Transitive Property for three things is illustrated in the above figure. Any of the following properties. This can be expressed as follows where a b and c are variables that represent the same number.

Transitive property is a generic notion. Transitive Property of Equality. This property can be applied to numbers algebraic expressions and various geometrical concepts like congruent angles triangles circles etc.

This is the transitive property at work. If whenever object A is related to B and object B is related to C then the relation at that end transitive provided object A is also related to C. Congruence has a transitive property.

Examples of transitive relations are less than for real numbers a b and b c implies a c and divisibility for integers a divides b and b divides c mean that a divides c. In Mathematics Transitive property of relationships is one for which objects of a similar nature may stand to each other. This is a property of equalityand inequalities.

In this situations we are told that apples are equal to bongos and that bongos are equal to cats. Click here for the full version of the transitive property of inequalities. In geometry we can apply the transitive property to similarity and congruence.

So if A5 for example then B and C must both also be 5 by the transitive property. The transitive property is a geometric rule the states if ab and bc then ac. It states that if two values are equal and either of those two values is equal to a third value that all the values must be equal.

One of the equivalence properties of equality. This is true ina foundational property ofmath because numbers are constant and both sides of the equals sign must be equal by definition. This is a property of equality and inequalities.

Transitive Property mathematics property of a mathematical relation such that if the relation holds between a and b and between b and c then it also exists between a and c. If a band b c then a c. If aband bc then ac.

You simply notice that 1 1 is present and 1 2 is present so transitivity demands that 1 2 be present. A relation has a transitive property if A B and B C implies that A C. The transitive property is also known as the transitive property of equality.

If giraffes have tall necks and Melman from the movie Madagascar is a giraffe then Melman has a long neck. Click here for the transitive property of equality. The transitive property of equality.

This property tells us that if we have two things that are equal to. Transitive Property for three segments or angles. If a b and b c then a c Another way to look at the transitive property is to say that if a is related to b by some rule and b is related to c by that.

Being parallel does also if consider a line being parallel to itself which we normally not do. If a band b c then a c. The fact that a b in your particular example doesnt change that.

In this situation a stands for apples b stands for bongos and c stands for cats. The transitive property of inequality states that if M is greater than N and N is greater than P then M is also greater than P. If a b and b c then a c.

If two segments or angles are each congruent to a third segment or angle then theyre congruent to each other. The transitive property meme comes from the transitive property of equality in mathematics. The transitive property states that.

In math if AB and BC then AC.

The Transitive And Substitution Properties Dummies

Transitive Property Of Equality Expii

Properties Of Equality

Transitive Property Of Congruence Substitution Property Of Equality Youtube

Triangle Congruence Proofs Ck 12 Foundation

Transitive Property Of Congruence Similar Triangles Tutors Com

The Transitive Property Of Congruence

Segment Proofs Diagram Quizlet

Transitive Property Of Congruence Similar Triangles Tutors Com

Geometry Two Column Proofs Of Angles Addition Substitution Transitive Property Youtube

Reflexive Property Of Equality Top Online General

Transitive Property Definition Geometry Page 1 Line 17qq Com

Transitive Property Of Congruence Similar Triangles Tutors Com

Substitution Property Of Equality Definition Examples Video Lesson Transcript Study Com

The Transitive Property Of Equality

Bell Work 1 Solve For Each Variable 2 Solve For Each Variable 3 And 4 Transitive Property Of Equality Definition Of Congruence Given Definition Of Congruence Ppt Download